Decide whether the equation is a trigonometric identity explain your reasoning cos^2x(1tan^2x)=1 secxtanx(1sin^2x)=sinx cos^2(2x)sin^2=0 ** Prove 1tan^2x=sec^2x using triangles Prove 1tan^2x=sec^2x using trianglesFree math problem solver answers your algebra, geometry, trigonometry, calculus, and statistics homework questions with stepbystep explanations, just like a math tutor0603 Prove 1tan^2x = sec^x anarkha1111 is waiting for your help Add your answer and earn pointsShow intermediate steps Learn how to solve trigonometric identities problems step by step online Prove the trigonometric identity (1cos (2x))/ (sin (2x)=tan (x) Apply the trigonometric identity 1\cos\left (2x\right)=2\sin\left (x\right)^2 Simplify \frac {2\sin\left (x\right)^2} {\sin\left (2x\right

Prove 1 Tan 2 Theta Sec 2 Theta Mathematics Stack Exchange
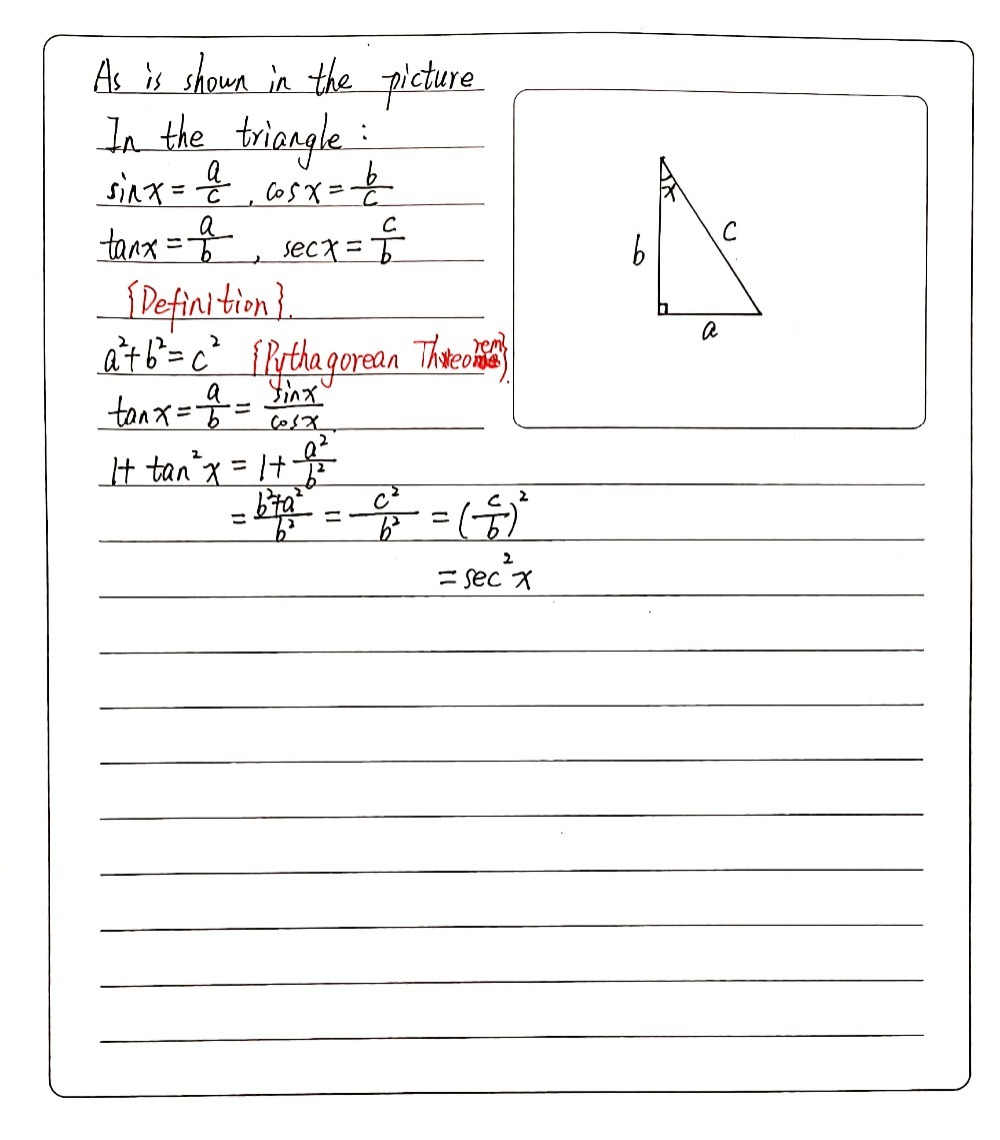
Prove 1+tan^2x=sec^2x using triangles
Prove 1+tan^2x=sec^2x using triangles-We are asked to prove {eq}(\sec x 1)(\sec x 1) = \tan^2x {/eq} When proving an identity, we only work with one side usually the messier one In this problem, we will work with the left sideUnlock this full stepbystep solution!



Q Tbn And9gctg1mv4zqwzzx9es1vyuwisok4cikumrujuyo2nyqlvyw5qkqqv Usqp Cau
I need to prove that $$1\tan x \tan 2x = \sec 2x$$ I started this by making sec 1/cos and using the double angle identity for that and it didn't work at all in any way ever Not sure why I can't do that, but something was wrong 1* (sec^2 (x) tan^2 (x)) Right side 12tan^2 (x) from the trig identity sec^2x tan^2x = 1 sec^2x tan^2x 2tan^2x = 12tan^2x simp lying this sec^2x tan^2x So right side now matches left side Ex 34, 8 Find the general solution of the equation sec2 2x = 1 – tan 2x sec2 2x = 1 – tan 2x 1 tan2 2x = 1 – tan2x tan2 2x tan2x = 1 – 1 tan2 2x tan2x = 0 tan 2x (tan2x 1) = 0 Hence We know that sec2 x = 1 tan2 x So, sec2 2x = 1 tan2 2x tan 2x = 0 ta
Tanx = t Sec^2 x dx= dt So now it is, 1/ (1t)^2 dt This integral is given by 1/1t and t= tanx So, it is cosx/cosx sinx tanx = t Sec^2 x dx= dt So now it is, 1/ (1t)^2 dt This integral is given by 1/1t and t= tanx So, it is cosx/cosx sinx Integral of the function \frac {\cos ^2 x} {1\tan x}Consider (tan^4x), (tan^4x = tan^2x (tan^2x) = tan^2x(sec^2x1) = sec^2x tan^2x tan^2x) Substitute the two back to (sec^4xsec^2x tan^2xtan^4x, and simplify it With the help of the identity sec^2xtan^2x = 1, you should be able to get the right sideSine The first trigonometric function;
25 sec^2x=1 tan^2x proof Sec^2x=1tan^2x proof Simplifying the LHS of the equation ,the LHS becomes Simplifying the above equation Using the identity The equation becomes Simplifying the above equation Using the identity mathsin2x=2sinxcosx,Prove the trigonometric identity `(tan^2(x))/(1tan^2(x)) = sin^2(x)` Substitute the trigonometric identity `tan^2(x) = sec^2(x)1` Note This is the same as `1 tan^2(x) = sec^2(x)`Since tanθ = sinθ cosθ and secθ = 1 cosθ , ⇒ tan2θ 1 = sec2θ Hence Proved cos(x − x) = cos2x sin2x = 1 then divide by cos2x to get the result above I've assumed the one of the trigonometric results d dθ(1 tan2θ) = 2tanθsec2θ d dθsec2θ = 2secθ(tanθsecθ) = 2tanθsec2θ Thus (1 tan2θ) − sec2θ is a constant
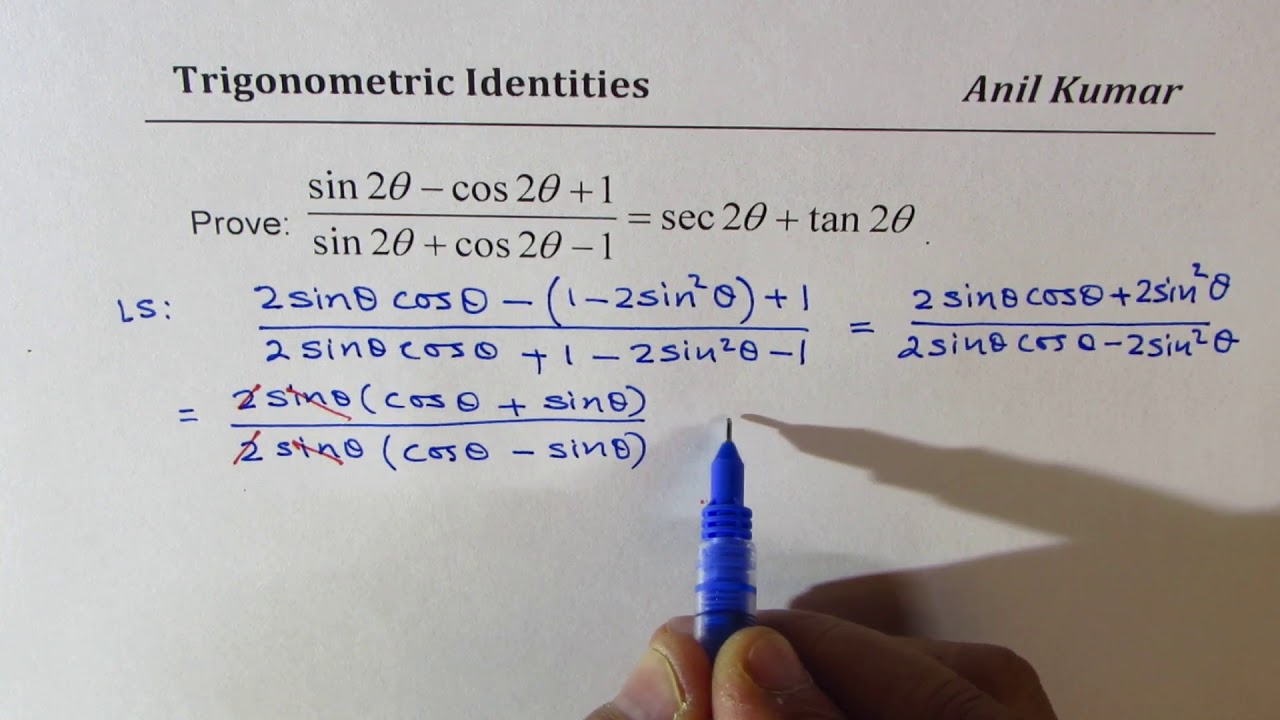



Trigonometric Identity In Double Angle Sin2x Cos2x 1 Sin2x Cos2x 1 Sec2x Tan2x Youtube




1 Given The Triangle Below Calculate The Following Chegg Com
Excellent application of Pythagorean Trig Identities email anilanilkhandelwal@gmailcom👍 Correct answer to the question Prove that tan^2x sec^2x=1 eeduanswerscomSecant, cosecant, and cotangent Prove that tan(x) = sin(x)/cos(x) Tangent;




Prove That Frac Mathit Sec 8x 1 Mathit Sec 4x 1 Frac Tan 8x Tan 2x Snapsolve




Integrate Sec 2x Method 1
Prove the identity (show your work please) tan(x − π/4)= (tan x − 1)/(tan x 1) Thank you! Divide both sides by cos2(x) to get cos2(x) cos2(x) sin2(x) cos2(x) = 1 cos2(x) which simplifies to 1 tan2(x) = sec2(x) Answer link Click here 👆 to get an answer to your question ️ prove this trigonometric equation;




Complete The Proof Of The Identity By Choosing The Gauthmath




How Many Can You Derive From First Principles Ppt Download
The given trigonometric identity is {eq}\frac{\tan \left(2x \right)}{1 \sec \left(2x \right)} = \tan \left(x \right) {/eq} Let us prove the trigonometric identitySec^4 x 2sec^2x=tan^4 x1 sec^2 x (sec^ x2)=tan^4 x1 tan^2 x1(tan^2 x12)=tan^4 x1 tan^2 x1(tan^2 x1)=tan^4 x1If 2x = sec A and 2/x = tan A prove that (x^2 1/x^2 ) = 1/4 Sarthaks eConnect Largest Online Education Community If 2x = sec A and 2/x = tan A prove that (x2 1/x2) = 1/4 Login




Sin2x Secx 1 Sec2x Sec2x 1 Brainly In



Solution Tan 2x Secx 1 1 Cosx Cosx Please And Thank You
Prove cot (2x)= (1tan^2 (x))/ (2tan (x)) Trigonometry Calculator Symbolab Identities Pythagorean Angle Sum/Difference Double Angle Multiple Angle Negative Angle Sum to ProductA=170 degree then prove that Tan A/2=1rot(1Tan^2 A)/Tan A math Prove that the equation Is an identity Sec^4x Tan^4x = Sec^2x Tan^2x PreCalc Help!!!Learn how to solve trigonometric identities problems step by step online Prove the trigonometric identity 1tan(x)^2=sec(x)^2 Applying the trigonometric identity \tan(x)^21=\sec(x)^2 Since both sides of the equality are equal, we have proven the identity
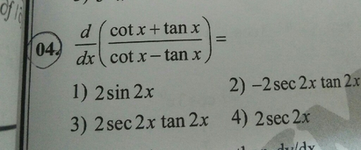



D Cotx Tanx Dx Cot X Tan X 1 2sin 2x 04 2 2 Sec 2x Tan 2 Scholr




Proof Tan 2 1 Sec 2 Youtube
A relationship between sine and cosine;Complete the proof of the identity by choosing the Rule that justifies each step cosx (1 tan 2x)secr To see a detailed description of a Rule in the Rule menu, select the corresponding question marProve the identity (1tanx) cotx=secx csex Note that each Statement must be based on a Rule chosen from the Rule menuGet answer sec ^(2) 2x =1tan 2x Apne doubts clear karein ab Whatsapp par bhi Try it now




Tan2x Sec2x ただの悪魔の画像
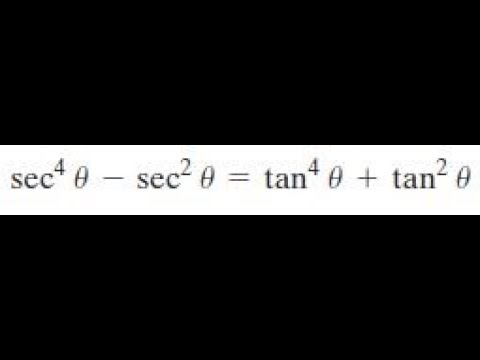



Sec 4 X Sec 2 X Tan 4 X Tan 2 X Youtube
Click here 👆 to get an answer to your question ️ If sec A = x 1/4x, prove that sec A tan A = 2x or 1/2x Explanation This is readily derived directly from the definition of the basic trigonometric functions sin and cos and Pythagoras's Theorem Confirming that the result is an identity Yes, sec2 − 1 = tan2x is an identity Prove that tan2(x) 1 = sec2(x) Prove that sin^2(x) cos^2(x) = 1;




1 Tan 2x 1 Tan 2 X 1 2cos 2 X Youtube




Sec X 1 Sec X 1 Tan 2 X Youtube
Question please help me prove this equation sec^4x2sec^2x=tan^4x1 Answer by vicgonzerx(31) (Show Source) You can put this solution on YOUR website! Here we will prove the problems on trigonometric identities As you know that the identity consists of two sides in equation, named Left Hand Side (abbreviated as LHS) and Right Hand Side (abbreviated as RHS)To prove the identity, sometimes we need to apply more fundamental identities, eg $\sin^2 x \cos^2 x = 1$ and use logical steps in order to lead oneTan^2x sec^2x = 1, baneenbilal8480 baneenbilal8480



Tan2x Sec2x ただの悪魔の画像




1 Prove That Cos 2x 2 Cos X 1 2 Prove That Chegg Com
`sin^2(x)cos^2(x)=1` Pythagorean theorem `tan^2(x)1=sec^2(x)` and `cot^2(x)1=csc^2(x)` `(sec^2(x)1)(csc^2(x)1)=2` `sec^2(x)csc^2(x) 2 = 2` `sec^2(x)csc^2(x) = 4` `1/(cos^2(x))1/(sin^2(xIdentities Proving Identities Trig Equations Trig Inequalities Evaluate Functions Simplify Statistics Arithmetic Mean Geometric Mean Quadratic Mean Median Mode Order Minimum Maximum Probability MidRange Range Standard Deviation Variance Lower Quartile Upper Quartile Interquartile Range Midhinge Standard Normal Distribution1 Tan 2x Sec 2x Proof bayern paris champions league bayern trikot 15 16 bayern spiel heute abend bayern vs dortmund 6 0 bayern münchen spielplan 19 bayern münchen sc freiburg bayern münchen training säbener straße bayern vs dortmund 18 19 Proof Tan 2 1 Sec 2 Youtube




How Do You Prove The Identity Tan 2x Secx 1 1 Cosx Cosx Socratic




What Would Be Steps In Proving That Tan2x Secx 1 1 Sec X Socratic
Prove that tan^2x sec^2x=1 Answers 3 Get Other questions on the subject Mathematics Mathematics, 14, klandry0 How long would it take to empty a pond that is 60x50x using a 1000 gallon pump Answers 3 continue Mathematics, 1730, iliketurtures In parallelogram abcd the ratio cos2x=(1tan^2x)/(1tan^2x) =(1tan^2x)/sec^2x =(1tan^2x)cos^x =cos^xsin^x =cos2xQ Prove Tan^2x1=sec^2x proof tan^2 x1=sec^2x So to get 1 on the other side of the equal sign wouldn't it be sec^2xtan^2x=1?Verify (1tan^2x)/(1cot^2x) = 1sec^2x Answered by a verified Math Tutor or Teacher We use cookies to give you the best possible experience on our website By continuing to use this site you consent to the use of cookies on your device as described inCan You Prove That Math Sec 2x Csc 2x 1 Tan 2x 1 Cot 2x Math Quora For more information and source, see on this link https//wwwquoracom/Canyouprovethatsec



Q Tbn And9gctg1mv4zqwzzx9es1vyuwisok4cikumrujuyo2nyqlvyw5qkqqv Usqp Cau



How Do We Prove That Tan2x 1 Sec2x Tanx Quora
Prove that (sin θ cos θ 1)/(sin θ cos θ 1) = 1/(sec θ tan θ), using the identity sec^2 θ = 1 tan^2 θ Find an answer to your question tan^2x 1 = sec^2x PROVE hopelafave hopelafave Math Secondary School answered Tan^2x 1 = sec^2x PROVE 1 See answer hopelafave is waiting for your helpClick here👆to get an answer to your question ️ Prove that inttan x sec ^2x √(1 tan^2x)dx = 1/3 ( 1 tan ^2x )^3/2




Prove That Tan 2 2x Tan 2 X1 Tan 2 2x Tan 2 X Tan 3x Tan X



Prove The Following I Sec 6 X Tan 6 X 1 3 Sec 2 X Tan 2 X Ii Tan8 Sec8 1 Sec8 1 Tan8 Sarthaks Econnect Largest Online Education Community
Prove $\frac{\tan{x}}{1\cot{x}} \frac{\cot{x}}{1\tan{x}}=\sec{x}\csc{x}1$ 2 Proving $(\sec^2x\tan^2x)(\csc^2x\cot^2x)=12\sec^2x\csc^2x$ and $\frac{\cos x}{1\tan x}\frac{\sin x}{1\cot x} = \sin x \cos x $Question i have to prove this identity 1/ tanx tanx = sec^2x/tanx i do have an idea on what to do but i get stuck every time this is what i did 1/ tanx tanx = sec^2x/tanx cot x 1/ cot x = Found 2 solutions by solve_for_x, Alan3354Prove that (tan^2x 1)cos2x=2sec^2x Pre Calculus Prove that the equation is an identity sec x/(sec x tan x)=sec^2 x sec x tan x trig prove that tan^2x sec^2x =1 precalculus For each of the following determine whether or not it is an identity and prove your result a cos(x)sec
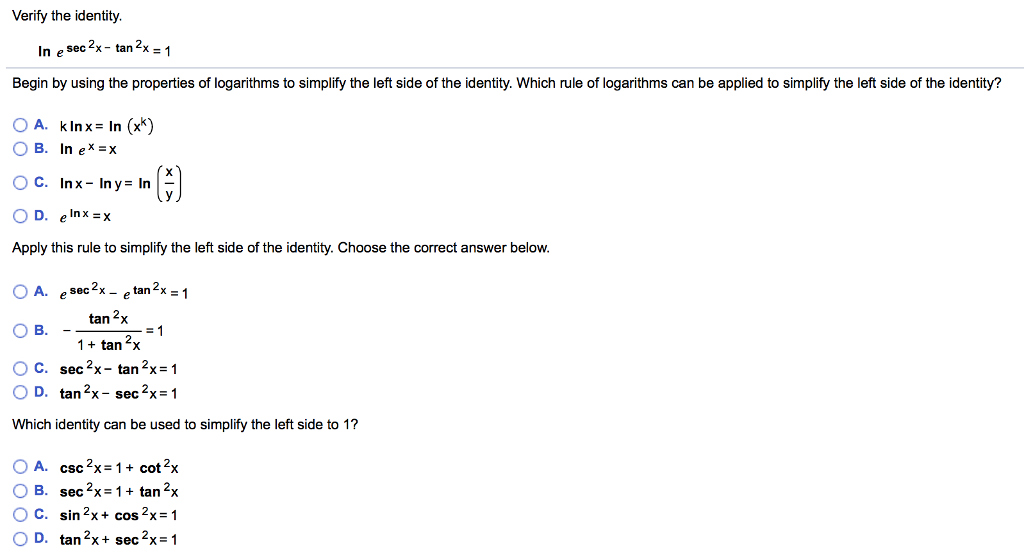



Verify The Identity In Esec 2x Tan 2x 1 Begin By Chegg Com




Qprove That Tan X 2 Tan 2x 4 Tan 4x 8 Cot 8x Cot X Mathematics Topperlearning Com Blpusg55
Click here👆to get an answer to your question ️ Prove that sec^6x tan^6x = 1 3sec^2x × tan^2xWell, using pythagarian identities we know that cos^2 u sin^2 u= tan^2 u ( and by deviding both sides by Cos^u) 1 tan^2 u= sec^2 u To Solve 2sec^2 x 1=12tan^2 x * Add 1 to both sides 2sec^2 x =22tan^2 x * Factor out the 2 sec^2 x= 1 tan^2 Ashwin Sreekumar, added an answer, on 23/5/15 Ashwin Sreekumar answered this 1tanx*tan2x = sec 2x LS =1 (sin x/cos x) (sin 2x/ cos 2x) =1 (sin x/cos x) (2sin x* cos x)/ cos 2x) =12sin^2 (x)/ (cos 2x) = {cos (2x) 2sin^2 (x)}/cos (2x) = {cos^2x sin^2 (x) 2sin^2 (x)/cos (2x)




Prove That Tex Frac Sin 2x Sec X 1 Frac Sec 2x Sec 2x 1 Tan Frac X 2 Tex Brainly In




Sec 6x Tan 6x 1 2 Tan 2x Sec 2x Important Difficult Trigonometric Identity Youtube
Isosceles and Equilateral Triangles; not sure how to start this one, i have tried it a few different ways and i still can't get it (1 tanx)^2 = sec^2x 2tanxUse the identities sec^2 (x) = tan^2 (x) 1 and csc^2 (x) = 1 cot^2 (x), both are derived from the pythagorean identity of 1 = sin^2 (x) cos^2 (x) by dividing through by either sin 2 or cos 2 2 View Entire Discussion (1 Comments)



Solved Explian The Proof For This Tan 2x 1 Tan X Co5 2x Solution Starting With The Left Side Sin 2x Sm X Step 1 Cos 2x Cos X Sin 2x Course Hero




If Tan 2 X Sec X A 0 Has Atleast One Solution Then Complete Set Of V Askiitians
Solve your math problems using our free math solver with stepbystep solutions Our math solver supports basic math, prealgebra, algebra, trigonometry, calculus and more




Warm Up Prove Sin 2 X Cos 2 X 1 This Is One Of 3 Pythagorean Identities That We Will Be Using In Ch 11 The Other 2 Are 1 Tan 2 X Sec 2 X Ppt Download




How Do You Prove Tan 2x Secx 1 1 Secx Socratic




How Do You Prove The Identities Cosx Secx Sinx Cscx Sec 2x Tan 2x Socratic




2 Prove The Following Trig Identities A Prove Tan Chegg Com




A Solution Of The Equation 1 Tan X 1 Tan X Sec 2x 2 Tan 2 0 Where X Lies In The Interval Pi2 Pi2 Is Given By
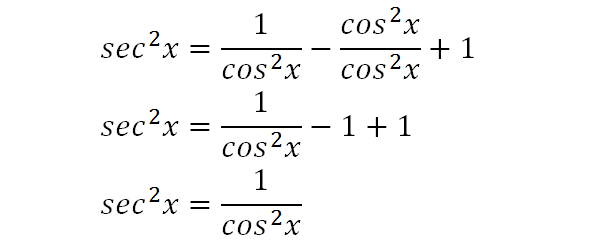



Integrate Sec 2x Method 2
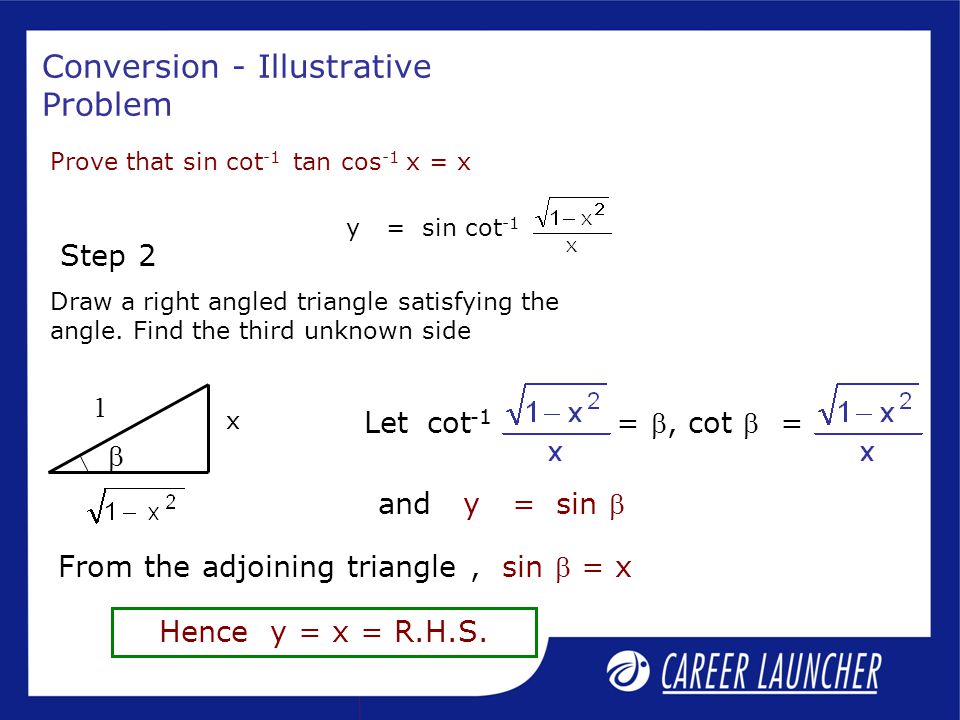



Mathematics Inverse Trigonometric Functions Session Ppt Download




How Does One Verify Cos 2x Sin 2x 1 Tan 2x Cos 2x Socratic
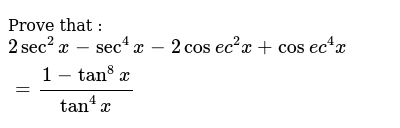



Prove That 2sec 2x Sec 4x 2cosec 2x Cosec 4x 1 Tan 8x Ta
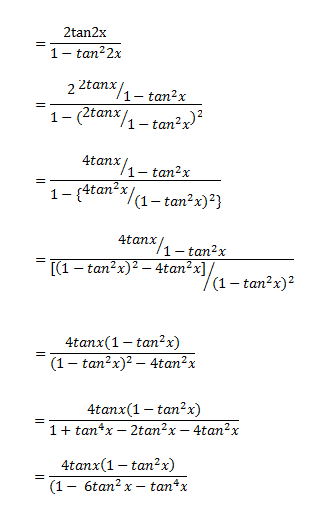



Trigonometry Functions Class Xi Exercise 3 3 Breath Math
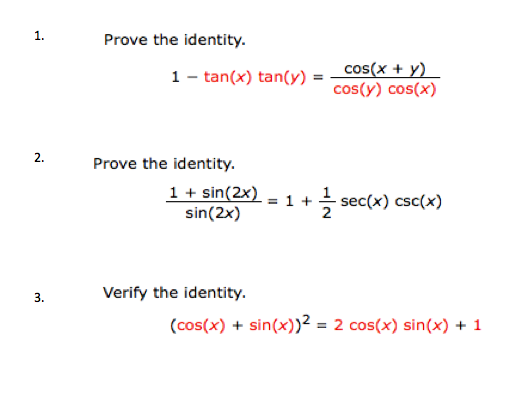



Prove The Identity 1 Tan X Tan Y Cos X Y Chegg Com




Answered 3 1 Tanx Tan 2x Sec 2x S Bartleby
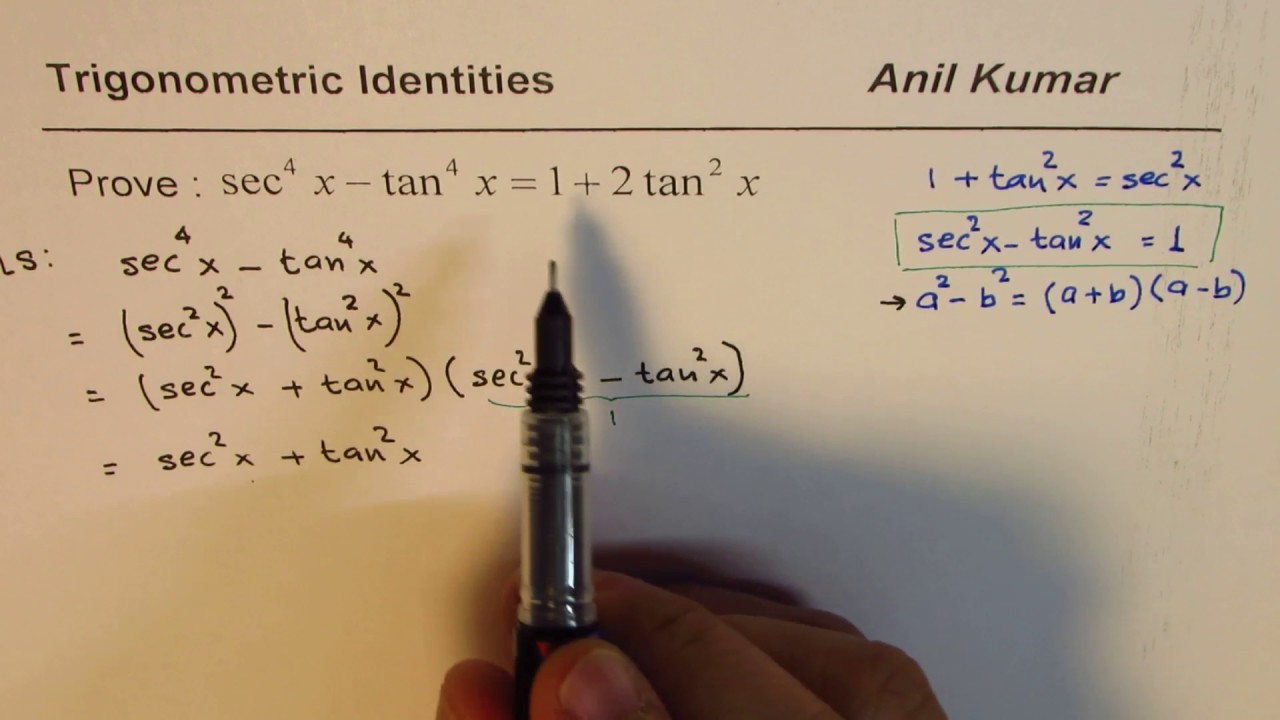



Trig Identity Sec 4x Tan 4x 1 2tan 2x Youtube



What Is The Formula Of Tan2x Quora




Is There Any Other Way To Establish This Trig Identity Frac Sec X 1 Tan X Frac Sin X 1 Cos X Mathematics Stack Exchange




Prove 1 Tan 2 Theta Sec 2 Theta Mathematics Stack Exchange



1




For 1 Through 4 Prove The Following Identities 1 Chegg Com




Trig Identity Tan X 1 Tan 2 X Sec X Cos X Csc X Sin X Proved From Both Sides Youtube



1
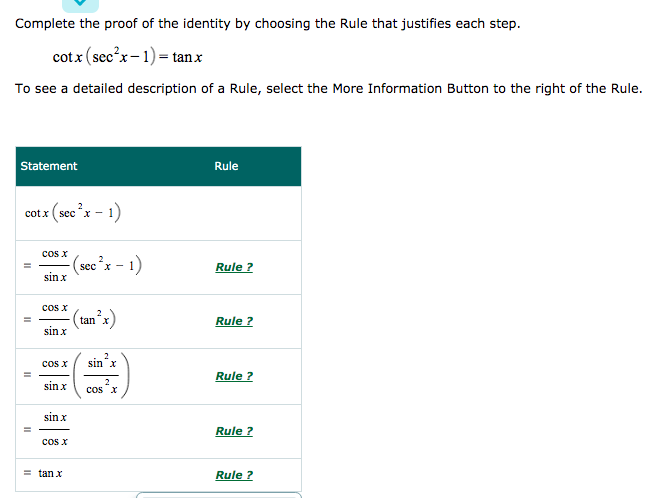



Complete The Proof Of The Identity By Choosing The Chegg Com




How Do You Simplify Sec 4x Tan 4x Sec 2x Tan 2x Socratic
.JPG)



Every Day I M Calculatin I D3 Unit Q Pythagorean Identities




How Do You Prove Tan 2x Secx 1 1 Secx Socratic
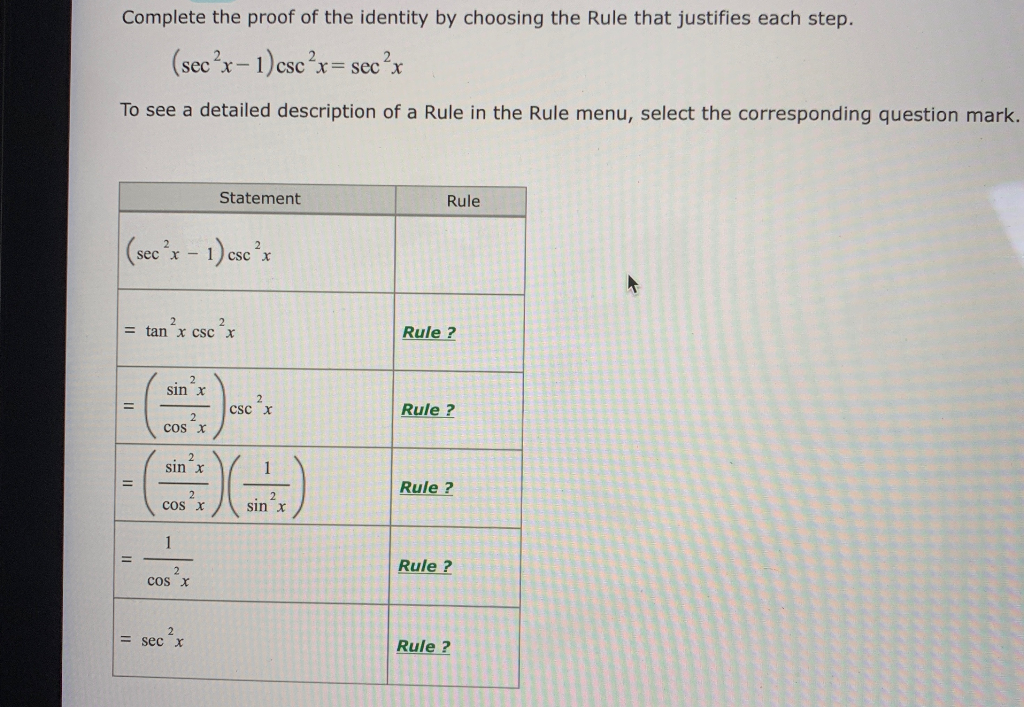



Complete The Proof Of The Identity By Choosing The Chegg Com



Solved Consider The Possible Identity Tan 2x Cos 2x 1 Cos 2x Sec 2x A State Any Non Permissible Values B Attempt To Verify Possible Identity Course Hero



Bestmaths Online Proof 4




6 A Given That Sin X R Find An Expressio Gauthmath
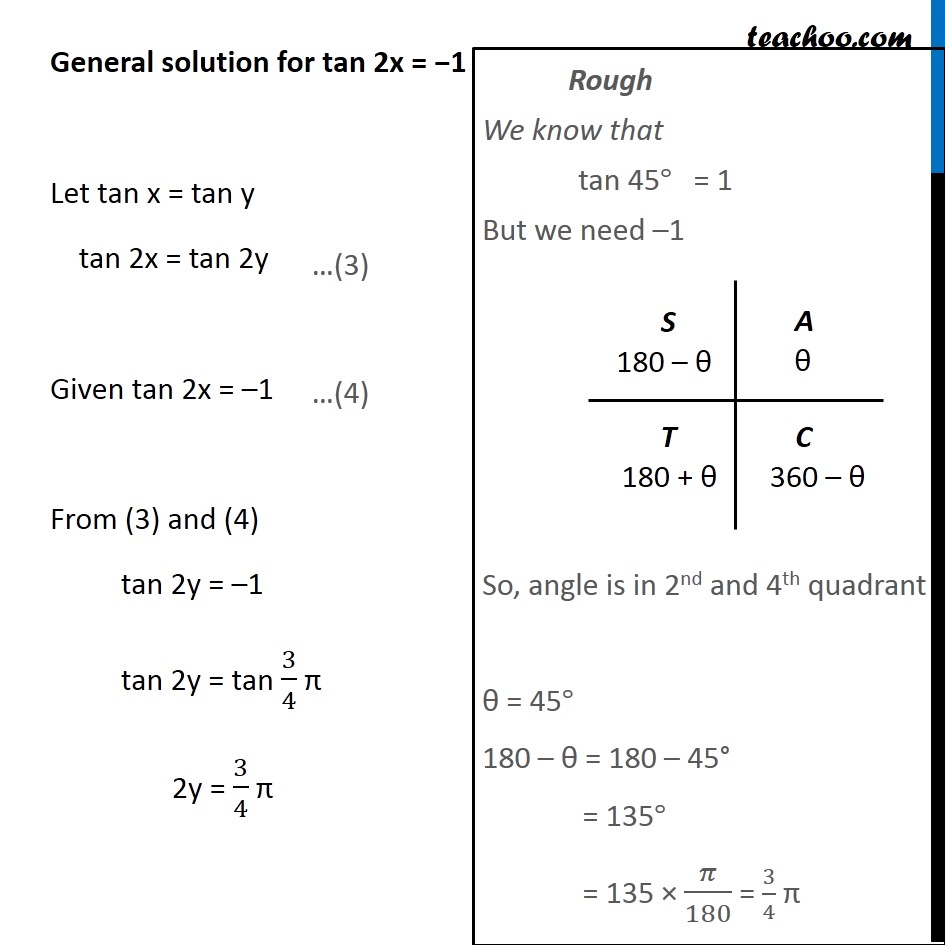



Ex 3 4 8 Find General Solution Of Sec 2 2x 1 Tan 2x Teachoo




Ex 3 3 23 Prove Tan 4x 4 Tan X 1 Tan2 X 1 6tan2x




Ex 3 4 8 Find General Solution Of Sec 2 2x 1 Tan 2x Teachoo
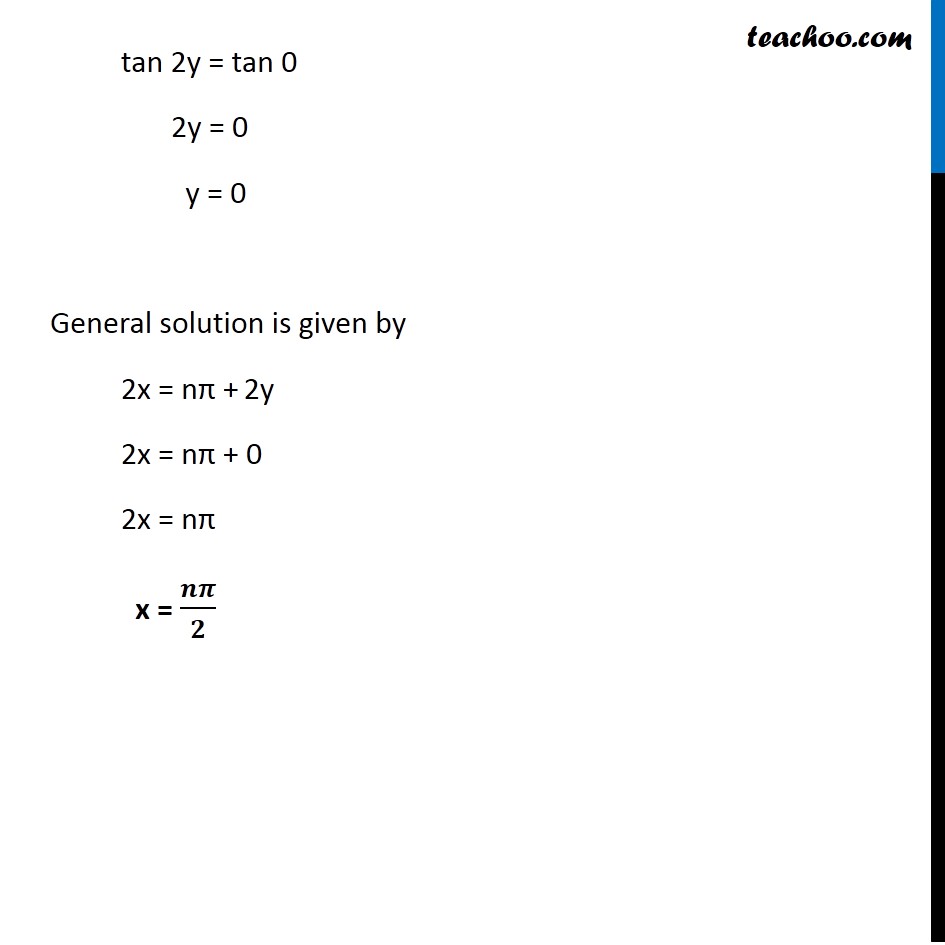



Ex 3 4 8 Find General Solution Of Sec 2 2x 1 Tan 2x Teachoo



Iit 1994 Prove That Sec2x Tan2x Tan Pi 4 X When X Lies Between 0 And Pi 4 Youtube



Answered Trigonometric Identities And Equations Bartleby



How To Prove D Dx Tanx Sec 2x Without Using Tanx Sinx Cosx And Instead Use Tan X H Tanx Tanh 1 Tanxtanh Quora




How To Prove That Math Frac Sec 8x 1 Sec 4x 1 Frac Tan 8x Tan 2x Math Quora



If Tan X T Then Tan 2x Sec 2x Is Equal To A 1 T 1 T Sarthaks Econnect Largest Online Education Community
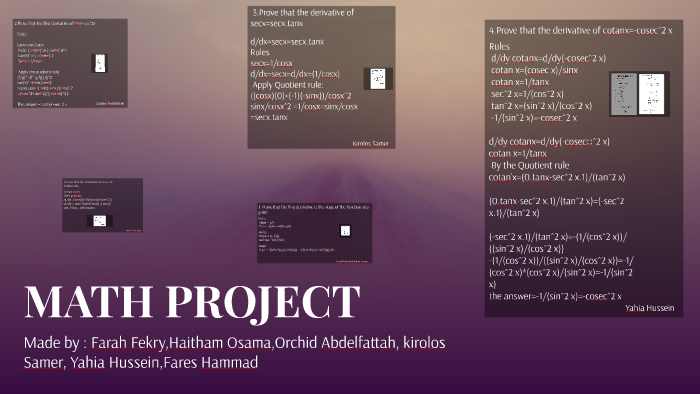



Math Project By Orchid Hassan On Prezi Next




Ex 3 4 8 Find General Solution Of Sec 2 2x 1 Tan 2x Teachoo
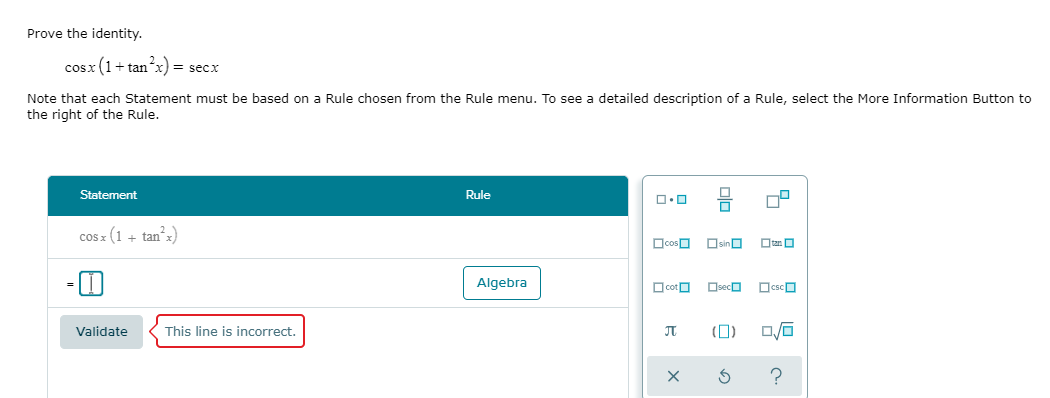



Prove The Identity Cosx 1 Tan2x Secx Note That Chegg Com
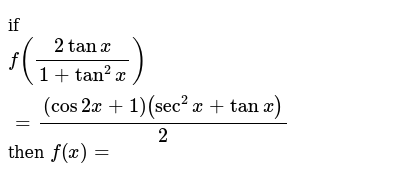



If F 2tanx 1 Tan 2x Cos2x 1 Sec 2x Tanx 2 Then F X



3




How To Show That Math Tan 2 X Sec 2 X 1 Math Quora
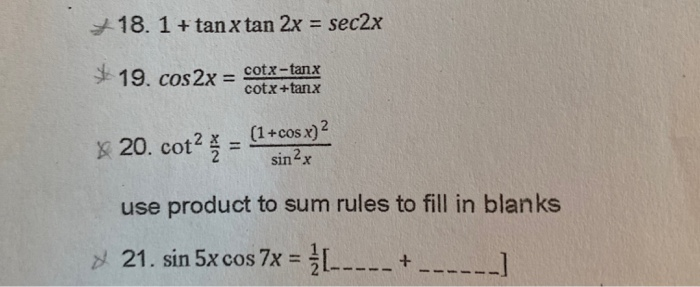



18 1 Tan X Tan 2x Sec2x 19 Cos2x Cotx Tank Chegg Com



What Trigonometric Identity Should I Use To Simplify 1 Tanx Secxtanx Secx Sec 2x 1 Tanx 2 The Answer Is Secx Tanx 1 1 Tanx 2 But Having Trouble Simplifying The Problem To Get The Answer Quora




Trig Identity Sec2x Minus Tan2x T10 Youtube




1 Tanx Tan X 2 Secx Tanx Cotx 2 1 Prove It Brainly In



Use A Similar Line Of Reasoning To Prove That 1 T Gauthmath



Prove The Identity Tan X 1 Cot X Cot X 1 Tan X Sec X Cosec X 1 Sarthaks Econnect Largest Online Education Community




2 Prove The Following Trig Identities A Prove Tan Chegg Com




Rd Sharma Solutions For Class 10 Chapter 6 Trigonometric Identities Exercise 6 1 Get Pdf




How Do You Verify 4tan 4 Tan 2x 3 Sec 2x 4tan 2x 3 Kinda Hard Please With All The Steps Thanks Socratic




Tangent Half Angle Formula Wikipedia



Solved Prove The Following Trig Identity Sec 2 X 2secx Cosx Cos 2 X Tan 2 X Sin 2 X Course Hero




Warm Up Prove Sin 2 X Cos 2 X 1 This Is One Of 3 Pythagorean Identities That We Will Be Using In Ch 11 The Other 2 Are 1 Tan 2 X Sec 2 X Ppt Download




1 Tan 2x 1 Cos 2x Sin 2x 2sin 4x 1 Sin 2x Trigonometric Identities Mcr3u Youtube
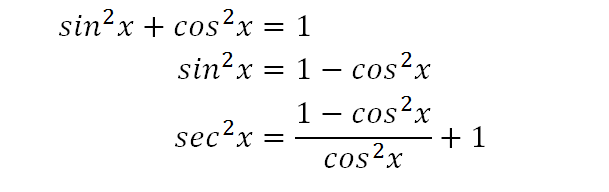



Integrate Sec 2x Method 2



How Do You Prove That Tanx Secx 1 Tanx Secx 1 1 Sinx Cosx Socratic




How Do You Simplify 1 Tan 2 X 1 Tan 2 X Socratic
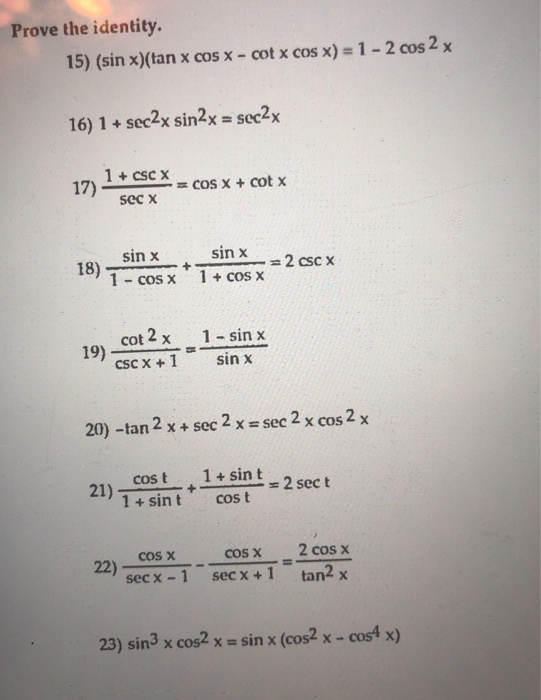



Prove The Identity 15 Sin X Tan X Cos X Cot X Chegg Com




If Sec A X 14x Then Prove That Sec A Tan A 2x Or 12x Maths Introduction To Trigonometry Meritnation Com




Find The General Solution For Each Of The Following Equations Sec2 2x 1 Tan 2x Mathematics Shaalaa Com




Verifying A Trigonometric Identity Tan 2 X 1 Sec X Sec X Youtube




Integrate Sec 2x Method 2




Simplify This Trig Function 1 Tan 2x Csc 2x Youtube
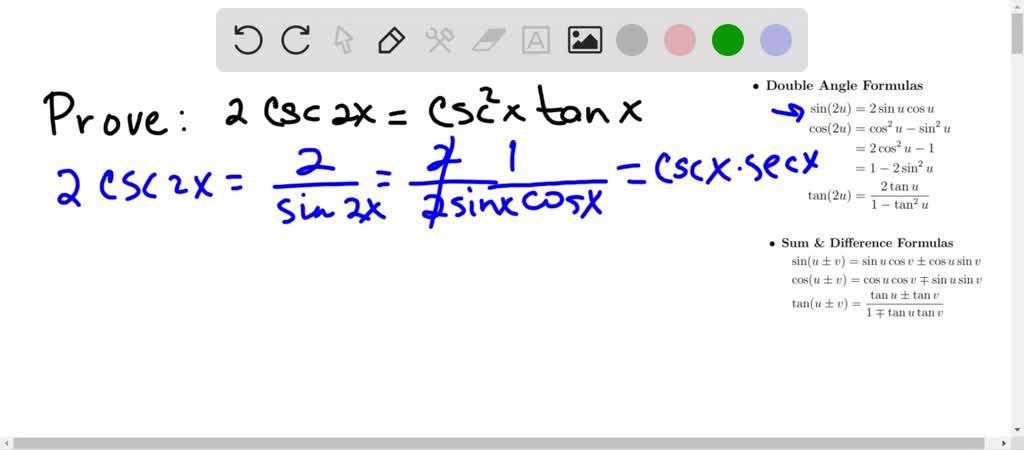



Solved In Exercises 15 22 Prove The Identity 2 Csc 2 X Csc 2 X Tan X




1 Tan1 1 Tan2 1 Tan3 1 Tan45 2 N Brainly In Ryad Antonio Ebooks En Ligne Gratuit




How To Show That Math Tan 2 X Sec 2 X 1 Math Quora




5 Prove Each Identity 3 4 4 Marks 2sines A Chegg Com



How To Prove That 1 Sin 2x 1 Cotx Cos 2x 1 Tanx Sinxcosx Using Trig Identities Quora
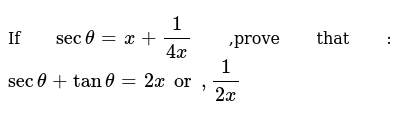



The Equation Sec 2x 1 Tan 2x Is An Identity True Or False



Prove Sin2x 2tanx 1 Tan 2x Socratic
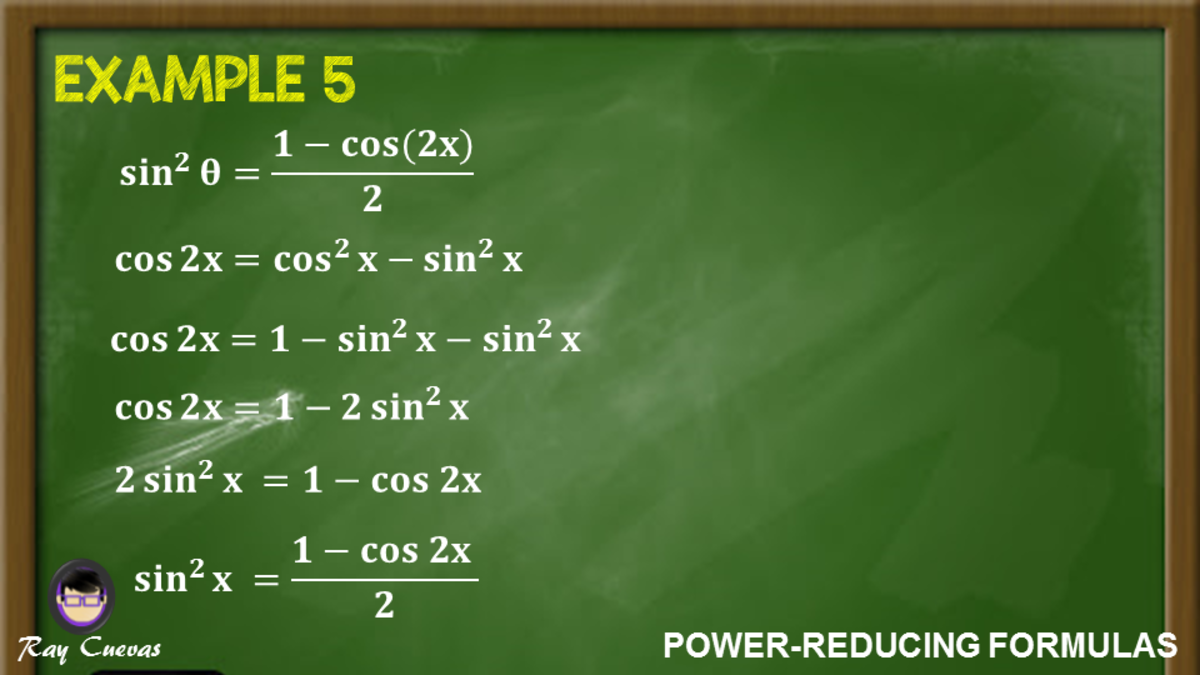



Power Reducing Formulas And How To Use Them With Examples Owlcation
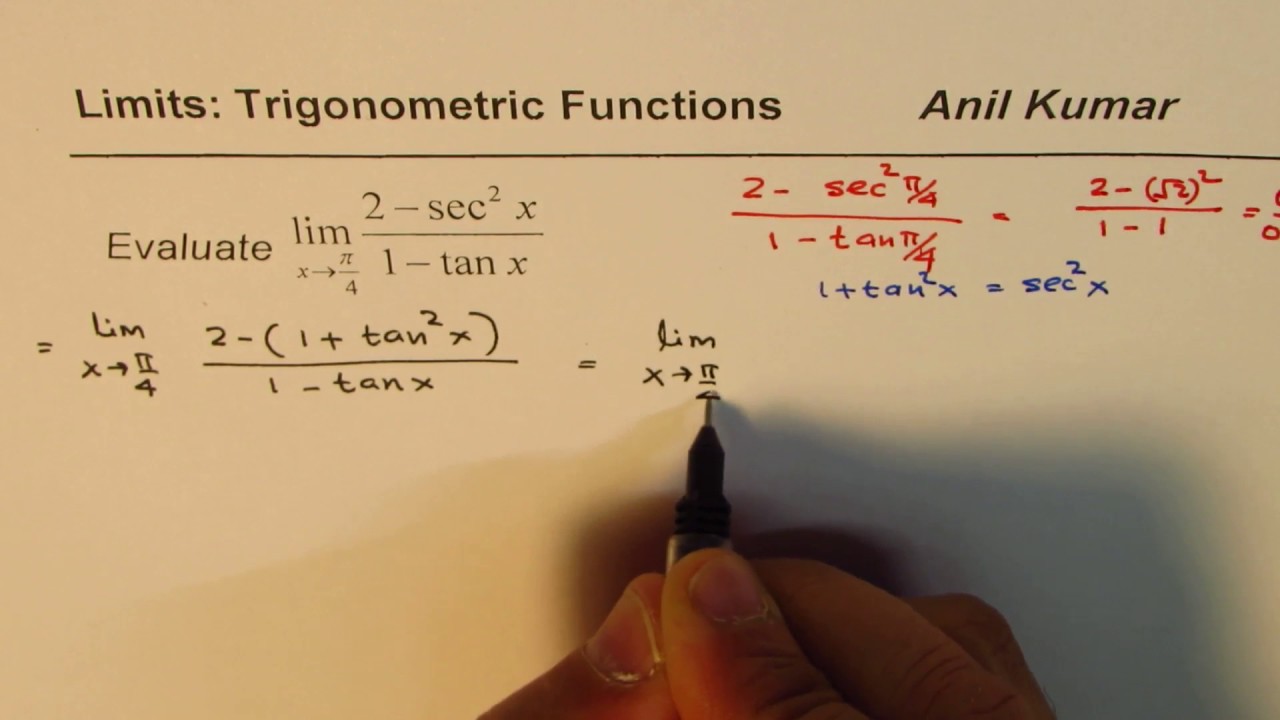



Limit Trigonometric Function 2 Sec 2x 1 Tan X Youtube



Tan2x Sec2x ただの悪魔の画像




Proving Trigonometric Identities Sec 2x Csc 2x Sec 2 X Csc 2



0 件のコメント:
コメントを投稿